
Books about science are popular. The physicist Brian Greene wrote a best seller untangling string theory and the cosmologist Hawkings gave us a lavishly illustrated coffee table tome, compressing the universe into a nutshell. Books about numbers are not that popular. But those who like to play with calculations and geometric diagrams will want to look at Mario Livio’s entertaining book about a very special numeral.
In The Golden Ratio Livio tells the story of Phi, “the world’s most astonishing number.” There are some numbers which turn up on special occasions and which have such curious characteristics that mathematicians eventually name them or, more precisely, symbolize them by a letter, usually from the Greek alphabet. Probably the best known numeral to be honored this way is the one you come up with when you divide the circumference of a circle by its diameter – the endless 3.14159…, the number called Pi, symbolized by the Greek π.
The Golden Ratio is 1.61803… and is symbolized by the Greek letter Φ, called Phi. Phi is not so well known as Pi, but it’s considerably more interesting and has been called the Golden Number, the Golden Ratio, the Golden Section, perhaps because of its esthetic value.
The first clear definition of Phi was given around 300 B.C. by Euclid, the Alexandrian who first formalized geometry. He pointed out that you can divide a straight line into two unequal parts, and if you divide it at precisely the right place the whole line will be to the larger segment as the larger segment is to the smaller segment. Or, to say it another way, the ratio of the whole line to the larger line segment is the same as the ratio of the larger segment to the smaller segment.
So what? Well, it turns out that the ratio of those line segments occurs in nature all the time and in remarkably beautiful forms. The lovely widening spiral of a chambered nautilus shell, the pattern of seeds in the face of a sunflower, the common arrangement of shoots on a plant stem and petals on a rose – all follow this number. Even a rectangular room whose sides are in the Golden Ratio makes a particularly harmonious volume. This handsome book has a lucid diagram or picture to illustrate such points on just about every other page.
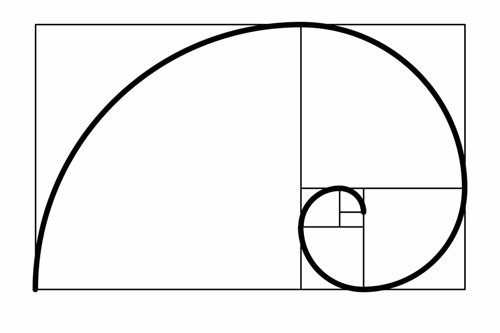
The nautilus is a mollusc of the cephalopod family and the photograph at the top of this piece is of a nautilus shell which has been sliced to reveal the shell’s inner chambers. Each of those chambers is 1.08 times larger than the previous chamber, and the shell expands in logarithmic fashion. It’s a beautiful work of nature, though perhaps not precisely a golden spiral. The spiral in the diagram below is a golden spiral. The ratio of the big rectangle enclosing the spiral has the same proportion to the large rectangle in the upper left of the diagram, as the upper left rectangle has to the smaller one to the right. Each of the rectangles has the same proportion or ratio in regard to the next smaller rectangle.
Mario Livio’s easy going narrative takes him to some strange places in the history of mathematics. Among the more interesting people we meet is the Greek philosopher and mathematician Pythagoras. The old professor wrote little or nothing but happily his students formed a society, the Pythagoreans, to preserve and carry on his work. Pythagoras, who was said to have a golden thigh, taught the doctrine of metempsychosis, that the soul is immortal and is reborn in human and animal incarnations. As you might guess, Pythagoreans were vegetarians. They were also crazy about geometry, and probably were the first to discover that the Golden Ratio is an unending problem in division, what mathematicians call an irrational number. And it’s irrational not because it’s insane, but because it can’t be produced by a ratio. Pi or π can be approximated by 22/7, but that’s only an approximation and in there’s no ratio that can get you an accurate Pi. Furthermore, irrational numbers don’t just go on endlessly, they also don’t repeat no matter how long you keep dividing.
Livio’s book is not a history of geometry or mathematics, definitely not a heavy text, but a remarkably lucid exploration of just about everything even remotely associated with Phi. It’s an exceptionally simple read. To be blunt, if there’s a weakness in The Golden Ratio it’s the book’s utter simplicity. People who are tempted to open a book on Phi do not need to be told that “Plato(428/427 B.C. —348/347 B.C.) was one of the most influential minds of ancient Greece and of western civilization in general.” And it’s plain silly to digress on such trivia as the first mention of Fibonacci’s name. (It occurred in a footnote in Histoire des Sciences Mathematique en Italie. Does that matter?)
Books about numbers tend to stay in print. So if you’re interested, or if you’re searching for a gift for that nerdy but nice friend who has numerical interests, take a look at books from earlier years, too. Probably the numeral with the most intriguing history is zero, a relative new comer in mathematical calculations. Robert Kaplan’s The Nothing That Is: A Natural History of Zero provides an entertaining chronicle of the great naught, as does Charles Seife’s rather supercharged narrative Zero: The biography of a Dangerous Idea.
You may have come across e, or 2.71828…., while playing around with logarithms, or perhaps you accidentally computed it while trying to calculate compound interest on a savings account. Eli Maor’s e: The Story of a Number is probably the best popular book on e. And, of course, there’s always Pi. Petr (or Peter) Beckmann’s short, concise A History of Pi is still available. Beckman is cranky author – mathematics attracts a special kind of crank – but his book is uncluttered and to the point. And David Blatner’s The Joy of Pi is a sane, wholesome and breezy work. Newer books have been written about some of these numbers since I wrote this review, but the hot little number for this one is Phi, irrational and beautiful, in Mario Livio’s The Golden Ratio.

